If an object is moving in any reference frame, then it has momentum in that frame.
The amount of momentum that an object has depends on two physical quantities: the mass and the velocity of the moving object. Momentum depends on velocity and it has a magnitude and a direction and is a vector quantity.
Momentum = Mass × Velocity
In physics, the symbol for momentum is usually denoted by a small p, so the above equation can be rewritten as:
where m is the mass and v the velocity. The SI unit of momentum is kilogram meters per second (kg m/s).
Impulse
A step function change in an object's momentum is known as an impulse. In physics, the symbol for impulse is usually denoted by a J or I.
The impulse (mass × change in velocity) = force applied × the time over which the force was applied.
Conservation of momentum and collisions
Momentum has the special property that, it is always conserved. Kinetic energy, on the other hand, is not conserved in collisions if they are inelastic. Since momentum is conserved it can be used to calculate unknown velocities following a collision.
A common problem in physics that requires the use of this fact is the collision of two particles. Since momentum is always conserved, the sum of the momentum before the collision must equal the sum of the momentum after the collision:
where the subscript i signifies initial, before the collision, and f signifies final, after the collision.
Elastic collisions
A collision between two pool balls is a good example of an almost totally elastic collision. In addition to momentum being conserved when the two balls collide, the sum of kinetic energy before a collision must equal the sum of kinetic energy after:
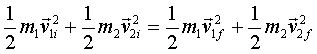
Since the 1/2 factor is common to all the terms, we can simplify the equation:
Changes in momentum
Although momentum is conserved within a closed system, individual parts of a system can undergo changes in momentum. In classical mechanics, an impulse changes the momentum of a body, and has the same units and dimensions as momentum. The SI unit of impulse is the same as for momentum (kg m/s). An impulse is calculated as the integral of force with respect to time.
where
I is the impulse, measured in kilogram meters per second
F is the force, measured in Newton (1 N = 1 kg•m/s2 )
t is the time duration, measured in seconds
Impulse as a change in momentum