When light is incident upon a medium of lesser index of refraction, the ray is bent away from the normal, so the exit angle is greater than the incident angle. Such reflection is commonly called "internal reflection". The exit angle will then approach 90° for some critical incident angle Θc, and for incident angles greater than the critical angle there will be total internal reflection.
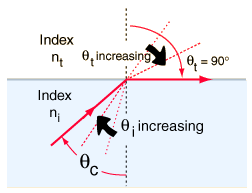
The critical angle can be calculated from Snell's law by setting the refraction angle equal to 90°.
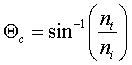
Condition for total reflection
For total internal reflection , Incident angle > Critical angle
Medium where light coming form must have higher index of refraction compared to second medium ( ni > nt)
For any angle of incidence less than the critical angle, part of the incident light will be transmitted and part will be reflected.
Variable definitions used in this explanation
n1 = ni = index of refraction of medium where light coming from
n2 = nt = index of refraction of the second medium
Θc = Critical angle
Θi = Θ1 = Incident angle
Θt = Θ2 = Transmittance angle = Exit angle
This video requires Flash Player 9